

Figure 1: Area represented by \int_{a}^{b}f(x)d\,x
Figure 1 shows the graph of a function, y=f(x), together with the area enclosed by this curve and the x-axis, which is represented by the definite integral

Figure 2: Volume formed by rotating the area from Figure 1 about the x-axis
Figure 2 shows the volume formed by rotating this area through 360 degrees about the x-axis.
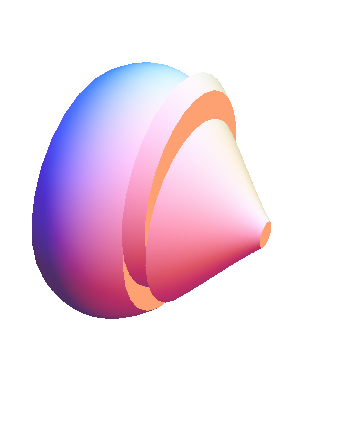
Figure 3: Slicing the volume into elemental discs
This volume can also be calculated using integration. We can think of it as being composed of thin slices, called elemental discs, as shown in Figure 3.

Figure 4: Each elemental disc is approximately cylindrical
As Figure 4 shows, each disc is approximately cylindrical. The radius of the cylinder is the value of the function, y. The thickness is the change in x, which we call \delta \,x. The volume of the cylinder is this approximately
The total volume is the sum of all these elemental volumes, which may be written
As we let the thickness of each slice, \delta \,x, approach zero, and the number of such slices gets larger and larger, this sum approaches the value of the integral
This is called the volume of revolution. For example, the volume of revolution of the curve y=x^{2}+1 about the x-axis between x=0 and x=1 is
which comes to
The volume of revolution about the y-axis of the area enclosed by a curve and the y-axis between y=a and y=b is, of course,

Figure 5: Volume of revolution about the y-axis